Question

The average age of a father and his four children is 12 years. The children are aged 3, 5, 6 and 10 years. Find the age of the father.​

Answer 1

Father=36 years old

Explanation:

5 people 4 children and a father

average age=12

add the ages

3+5+6+10=24

24+12=36

check the work

36 +24=60

60/5=12

answer=36

Answer 2

36 years old

Explanation:

The average age of father and four children can be expressed in mathematics as:

`\displaystyle \large{(f+c_1+c_2+c_3+c_4)/(5)}`

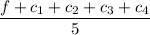
The above expression is what you call average value or mostly known as mean - the mean value is sum of all data values divided by number of data values. The formula reference for mean/average value is:

`\displaystyle \large{(x_1+x_2+x_3+...+x_n)/(n) = (1)/(n) \sum_(i=1)^n x_i}`

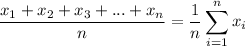
Determine or let the following be:

• f = father’s age
• c1 = first child’s age
• c2 = second child’s age
• c3 = third child’s age
• c4 = fourth child’s age

It is also given in the question that children’s ages are 3, 5, 6 and 10. Hence:

`\displaystyle \large{(f+3+5+6+10)/(5) = 12}`

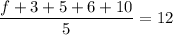
From above, it is also given that average of father’s age and children’s age is 12 years old - that now gives us an equation. What we have to do now is to solve for f.

First, multiply 5 both sides to get rid of denominator.

`\displaystyle \large{(f+3+5+6+10)/(5) \cdot 5 = 12 \cdot 5}\\\\\displaystyle \large{f+3+5+6+10=60}`

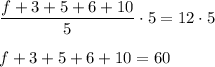
Evaluate 3+5+6+10 which equals 24.

`\displaystyle \large{f+24=60}`

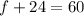
Next, subtract both sides by 24 to isolate f-term.

`\displaystyle \large{f+24-24=60-24}\\\\\displaystyle \large{f=36}`

Finally, we have received the value of term which is f = 36. We know that the term represents father’s age and therefore, father’s age is 36 years old!

If you have any doubts, please let me know in the comment!