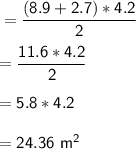Answer:
Explanation:
Area of shapes
a) Triangle
base = b = 7 km
altitude or height = h = 3.8 km
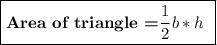

b) Rectangle:
length = l = 8.2 cm
width = w = 7 cm
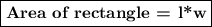
= 8.2 * 7
= 57.4 cm²
c) Parallelogram
base = b = 9.1 km
height = h = 5 km
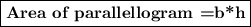
= 9.1 * 5
= 45.5 km²
d) Trapezium
Parallel sides a = 8.9 m & b = 2.7 m
height = h = 4.2 m