Question

A 1.6 kg block slides with a speed of .95 m/s on a frictionless, horizontal surface until it encounters a spring with a spring constant of 902 N/m.

A) How much does the spring compress when the block comes to rest?

B) When the block has compressed the spring 1.8 cm how fast is the block moving?

Answer 1

(a) the compression of the spring when the block comes to rest is 4cm

(b) speed of the block is 0.427 m/s

Step-by-step explanation:

Given;

mass of the block, m = 1.6 kg

spring constant, k = 902 N/m

initial speed of the block, v₀ = 0.95 m/s

(a) the compression of the spring when the block comes to rest

when the block comes to rest, the final speed, vf = 0

Apply the law of conservation of energy;

¹/₂kx² = ¹/₂mv₀²

kx² = mv₀²

`x = \sqrt{(mv_0^2)/(k) } \\\\x = \sqrt{(1.6(0.95)^2)/(902) }\\\\x = 0.040 \ m`

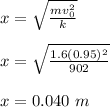
x = 4 cm

(b) speed of the block

Apply the law of conservation of energy;

¹/₂mv² = ¹/₂kx²

mv² = kx²

`v = \sqrt{(kx^2)/(m)}\\\\v = \sqrt{((902)(0.018)^2)/(1.6)}\\\\v = 0.427 \ m/s`