Question

Two hoses A and B together fill a swimming pool in two hours. A does it by herself in three hours less than B. Calculate how many hours it takes each to fill the pool.

Answer 1

If hose A takes x hours to fill the pool, hose B will take x+3 hours to fill the pool. So, each hour, A will fill
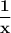`\bf{(1)/(x)}` parts of the pool and B will fill
`\bf{(1)/(x+3)}` parts. Since using both hoses fills the pool completely, you have to:

`\large\displaystyle\text{$\begin{gathered}\sf \bf{ 1= (1)/(x)+(1)/(x)+(1)/(x+3)+(1)/(x+3) } \end{gathered}$}\\\large\displaystyle\text{$\begin{gathered}\sf \bf{ \ \ \ =(2)/(x)+(2)/(x+3) } \end{gathered}$}\\\large\displaystyle\text{$\begin{gathered}\sf \bf{ \ \ \ =(2x+2x+6)/(x(x+3)) } \end{gathered}$}`

`\large\displaystyle\text{$\begin{gathered}\sf \bf{ x^2+3x=4x+6\ \Longrightarrow\ \ 0=x^2-x-6=(x-3)(x+2) } \end{gathered}$}`

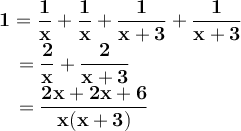
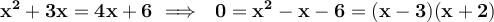
Hose A takes 3 hours to fill the pool and Hose B takes 6 hours.