Question

What is a simpler form of the radical expression?

SOMEONE PLEASE HELP!!

Answer 1

Choice B

Explanation:

Given radical expression:

`\sqrt[4]{1296 {x}^(16) {y}^(12) }`

To Find:

The Simpler form of this expression

Soln:

`= \sqrt[4]{1296 {x}^(16) {y}^(12) }`

We could re-write the given expression, according to the law of exponents:

`= \tt \sqrt[4]{(6x {}^(4)y {}^(3)) {}^(4) }`

Now we need to bring terms out of the radical as:

`\tt = | 6x { }^(4) y {}^(3) |`

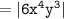
Bring out 6x^4 from the absolute & put y^3 only in it:

`\tt = 6x {}^(4) |y {}^(3) |`

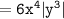
Choice B is accurate.