Question

Question 7

A thick uniform rubber rope of density 0.7 gcm-3 and Young Modulus 5 x 106 Nm 2 has a length 6.7 m. when
hung from the ceiling of the room, calculate the increase in length due to its own weight ?

O A. 35.79 x 10-2 m
O B. 43.81 x 10-2 m
OC.52.34 x 10-2 m
O D.61.65 x 10-2m
​

Answer 1

Increase in length, e = 61.59 * 10⁻²m

The closest value from the options is option D. 61.65 x 10-2m

Step-by-step explanation:

Young Modulus = stress/strain = (F/A)(/e/L) = F * L/A * e

where F = force; L = original length; A = area; e = increase in length

making e subject of formula;
`e = F * L/Y * A`

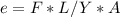
Also force, F = mass * acceleration due to gravity;

mass = density * volume = density * area * length = ρ * A * L

Therefore, force, F = ρ * A * L * g

e = ρ *A * L* L * g/Y * A = ρgL²/Y

density = 0.7 gcm⁻³ = 0.7 * 10³ kgm⁻³; g = 9.8 ms⁻²

e = {0.7 * 10³ kgm⁻³ * (6.7 m)² * 9.8 ms⁻²}/5 * 10⁶ Nm²

e = 61.59 * 10⁻² m