Answer:
The displacement is about 4.47 miles
Step-by-step explanation:
Displacement
Is the vector that represents the difference in positions that an object has moved on.
If the object was at position
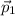 and ended up in the position
and ended up in the position
 , then the displacement is:
, then the displacement is:
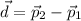
The magnitude of d is the length of the vector. Given its rectangular coordinates (dx,dy), then the magnitude is:
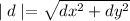
If you walk 2 miles east from the origin, your new position is (2,0). When you now walk 4 miles north, your final position is (2,4).
The displacement is:
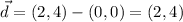
And its magnitude is:
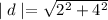
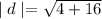
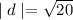
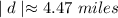
The displacement is about 4.47 miles