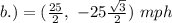Answer:
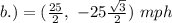
Explanation:
Given;
velocity of the diver, v = 25 mph
direction of his dive, θ = 60°
The vertical component of the velocity is given by;
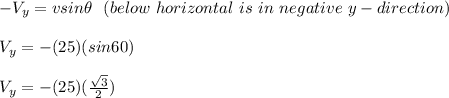
The horizontal component of the velocity is given by;
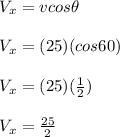
Therefore, the component form of the velocity vector is given by;
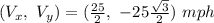
correct option =