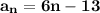Answer:

Explanation:
Arithmetic Sequences
The arithmetic sequences can be identified because each term is obtained by adding or subtracting a fixed number to the previous term. That number is called the common difference.
The equation to calculate the nth term of an arithmetic sequence is:

Here a1 is the first term and r is the common difference.
The given sequence is:
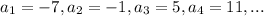
We can find the common difference by subtracting successive terms:

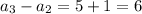
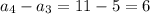
Since all the differences are equal, r=6. Thus, the general term is:
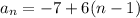
Operating:
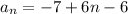

The nth term is: