Question

wheelchair ramp forms 20° angle with the ground. If the length of the ramp to the porch is 21.5 feet, how high (H) off the ground is the porch?

Answer 1

The porch is 7.35 feet off the ground

Explanation:

Trigonometric Ratios

The ramp, the height of the porch, and the distance from the base of both form a right triangle where trigonometric ratios can be applied.

The angle of 20° has the height of the porch H as the opposite side, and the hypotenuse is the length of the ramp.

The sine is defined as:

`\displaystyle \sin\theta=\frac{\text{opposite leg}}{\text{hypotenuse}}`

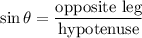
For our problem:

`\displaystyle \sin 20^\circ=(H)/(21.5)`

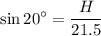
Solving for H:

`H=21.5\cdot \sin 20^\circ`

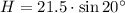
Find the sine of 20° by using a calculator:

`H=21.5\cdot 0.342`

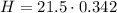
H=7.35 feet

The porch is 7.35 feet off the ground