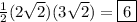Question

What is the area of a triangle whose vertices are X(1, 1), Y(3, -1), and Z(4,4)?

Answer 1

Explanation:

Note that in this diagram, point A represents point X, point B represents point Y, and point C represents point Z.

From the diagram, it appears as if
`\overline{XZ} \perp \overline{XY}`. To determine if this is the case, we can find the slopes of both segments.

`m_{\overline{XZ}}=(4-1)/(4-1)=1\\m_{\overline{XY}}=(-1-1)/(3-1)=-1`

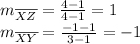
Since these slopes are negative reciprocals, we know that they are perpendicular.

This means we can use the formula
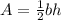`A=(1)/(2)bh`, where b is the base (XY) and h is the height (XZ).

• Note these are interchangeable.

Using the distance formula,

`XY=\sqrt{(3-1)^(2)+(-1-1)^(2)}=2√(2)\\XZ=\sqrt{(4-1)^(2)+(4-1)^(2)}=3√(2)`

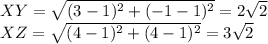
This means the area is
`(1)/(2)(2√(2))(3√(2))=\boxed{6}`