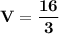Answer:
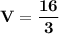
Explanation:
From the information given:
A = (4,0,0) B = (0,4,0) C = (0,0,1) D = (4,4,1)
 = (0,4,0) - (4,0,0) = (-4, 4, 0)
= (0,4,0) - (4,0,0) = (-4, 4, 0)
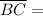 (0,0,1) - (0,4,0) = (0, -4, 1)
(0,0,1) - (0,4,0) = (0, -4, 1)
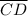 = (4,4,1) - (0,0,1) = (4, 4, 0)
= (4,4,1) - (0,0,1) = (4, 4, 0)
The Volume of the tetrahedron
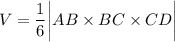
The Volume of the tetrahedron
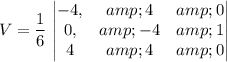
![V = (1)/(6)\bigg [ -4(-4 *0 - 1*4) -4(0*0-1*4) +0(0*4--4*4) \bigg ]](https://img.qammunity.org/2021/formulas/mathematics/college/as3pwwlczplogp3lbbq2kny7kt3bhdzfcf.png)
![V = (1)/(6)\bigg [ -4(0 - 4) -4(0-4) +0(0+16) \bigg ]](https://img.qammunity.org/2021/formulas/mathematics/college/9doesha4k0g0t359pwehq6yj2uis1zv285.png)
![V = (1)/(6)\bigg [ 16 + 16 +0 \bigg ]](https://img.qammunity.org/2021/formulas/mathematics/college/b8snzuvaoa7dgdx9ikt0fcwd6rqljxpksc.png)
![V = (1)/(6)\bigg [ 32 \bigg ]](https://img.qammunity.org/2021/formulas/mathematics/college/hrd1smgxomsfk4zzqowb1zp95h4korzkmu.png)