Answer:
Answer: -1
Explanation:
The Polynomial Remainder Theorem
It states that the remainder of the division of a polynomial f(x) by (x-r) is equal to f(r).
We have the polynomial:
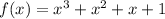
And we need to determine if x=1 and/or x=-1 are zeros of the polynomial.
Considering the polynomial remainder theorem, if we try any value for x, and the remainder is zero, then that value of x is a root or zero of the polynomial.
Find:
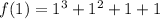
f(1)=4
Thus, x=1 is not a zero of f(x)
Now, find:


Thus, x=-1 is a zero of f(x)
Answer: -1