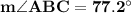The text of the question is not visible in the answering window. I'll reproduce it here:
BD bisects <ABC.
m <ABD= 2.5x + 8.6
m<CBD = 3.5x - 3.4
Find m<ABC
Answer:
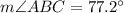
Explanation:
We have an angle ABC and a line BD bisecting it.
If an angle is bisected, then the two formed angles are congruent, that is

Substituting the algebraic expressions for both angles:
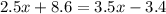
Subtracting 8.6 and 3.5x:
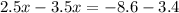
Operating:
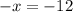
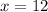
The two angles are:

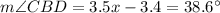
As expected, both angles have the same measure.
The measure of the total angle ABC is twice any of those: