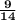Answer:
The required probability is
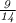
Explanation:
Probability: Rule of Addition
The probability that Event A or Event B occurs is equal to the probability that Event A occurs plus the probability that Event B occurs minus the probability that both Events A and B occur. It can be calculated as follows:
P(A ∪ B) = P(A) + P(B) - P(A ∩ B)
There are 14 types of crackers the company sells, numbered from 1 to 14. The sample space for purchasing one cracker is:
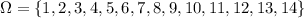
There are n=14 possible choices.
The favorable cases for a cracker with an odd number are:
A = {1,3,5,7,9,11,13}
There are 7 favorable cases.
The favorable cases for a cracker with a number greater than 11 are:
B = {12,13,14}
There are 3 favorable cases.
The cases which are common to both events are:
A ∩ B= {13}
There is 1 case.
The probability of each event is:

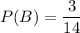
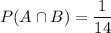
Therefore:
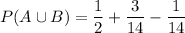
Adding the fractions:
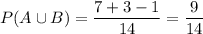
The required probability is