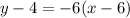Question

Find a​ point-slope equation of the line having the given slope and containing the given point.

m=−6​; ​(6​,4​)

Answer 1

`\boxed {y - 4 = -6(x - 6)}`

Explanation:

Since you already have the given slope and the given point, use the Point-Slope Formula to make it a Point-Slope Form:

`y - y_(1) = m(x - x_(1))`

Slope:
`m`

First Point:
`(x_(1), y_(1))`

-Substitute both the given slope and the given point:

Slope:
`-6`

First Point:
`(6,4)`

`\boxed {y - 4 = -6(x - 6)}` (Substituted in Point-Slope Form)

Answer 2

y-4 = -6(x-6)

Explanation:

Pre-Solving

Given

We are given that a line has a slope of -6 and a point of (6, 4).

We want to find the equation of this line in point-slope form.

Formulas

Point-slope form is given as
`y-y_1=m(x-x_1)`, where m is the slope and
`(x_1, y_1)` is a point.

Solving

Substitute the value of m (which is -6) into the formula for point-slope form.

`y-y_1=-6(x-x_1)`

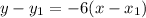
Now, substitute 6 and 4 as
`x_1` and
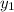`y_1` respectively.

`y-4=-6(x-6)`