Question

Find the magnitude of the gravitational force (in N) between a planet with mass 6.00 X 10^24 kg and its moon, with mass 2.50 X 10^22 kg, if the average distance between their centers is 2.70 X 10^8 m. What is the moon's acceleration (in m/s2) toward the planet? (Enter the magnitude.) What is the planet's acceleration (in m/s2) toward the moon? (Enter the magnitude.)

Answer 1

Magnitude of gravitational force between this planet and its moon: approximately
`1.37 * 10^(20)\; \rm N`.

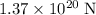Acceleration of this moon towards the planet: approximately
`5.49 * 10^(-3)\; \rm m \cdot s^(-2)`.

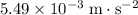Acceleration of this planet towards its moon: approximately
`2.29 * 10^(-5)\; \rm m \cdot s^(-2)`.

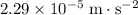Step-by-step explanation:

Look up the gravitational constant,
`G`:

`G \approx 6.67 * 10^(-11)\; \rm m^3\cdot kg^(-1) \cdot s^(-2)`.

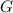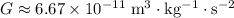Assume that both this planet and its moon are spheres of uniform density. When studying the gravitational interaction between this planet and its moon, this assumption allows them to be considered as two point masses.

The formula for the size of gravitational force between two point masses
`m_1` and
`m_2` with a distance of
`r` in between is:

`\displaystyle F = (G \cdot m_1 \cdot m_2)/(r^2)`,

where
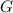`G` is the gravitational constant.

Let
`m_1` and
`m_2` denote the mass of this planet and its moon, respectively.

Calculate the size of gravitational force between this planet and its moon:

`\begin{aligned} F &= (G \cdot m_1 \cdot m_2)/(r^2) \\ &\approx \frac{6.67 * 10^(-11)\; \rm m^3 \cdot kg^(-1) \cdot s^(-2) * 6.00 * 10^(24)\; \rm kg * 2.50 * 10^(22)\; \rm kg}{{\left(2.70 * 10^(8)\; \rm m\right)}^2} \\ &\approx 1.37 * 10^(20)\; \rm N\end{aligned}`.

Assume that other than the gravitational force between this planet and its moon, all other forces (e.g., gravitational force between this planet and the star) are negligible. The magnitude of the net force on the planet and on the moon should both be approximately
`1.37 * 10^(20)\; \rm N`.

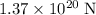Apply Newton's Second Law of motion to find the acceleration of this planet and its moon:

`\displaystyle \text{acceleration} = \frac{\text{net force}}{\text{mass}}`.

For this moon:

`\displaystyle (1.37 * 10^(20)\; \rm N)/(2.50 * 10^(22)\; \rm kg) \approx 5.49* 10^(-3)\; \rm m \cdot s^(-2)`.

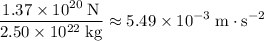For this planet:

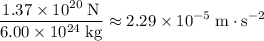`\displaystyle (1.37 * 10^(20)\; \rm N)/(6.00 * 10^(24)\; \rm kg) \approx 2.29 * 10^(-5)\; \rm m \cdot s^(-2)`.