Question

For a certain rectangular region, the ratio of its length to its width is 6 to 4. If the width of the

rectangular region increases by 11 units, how must the length change to maintain this ratio?

Answer 1

`22.5-6=16.5\ \text{units}`

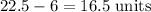
Step-by-step explanation:

The ratio of length and width is
`(6)/(4)`

Now the width increases by 11 units. Let the length be x units

The ratio of the sides will be the same for the new rectangle, so we get

`(6)/(4)=(x)/(4+11)\\\Rightarrow (6)/(4)=(x)/(15)\\\Rightarrow x=(6)/(4)* 15\\\Rightarrow x=22.5\ \text{units}`

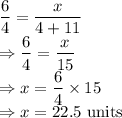
The length of the new rectangle would be
`22.5\ \text{units}`

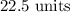
Increase in length is
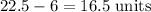`22.5-6=16.5\ \text{units}`.