Question

The Umbrella Corporation has found that in any given month, they sell fewer umbrella when the cost of the umbrella is higher. When the price of the umbrella is $10, they sell on average 18,000 umbrellas. When the price of the umbrellas is $16, they sell on average 9,000 umbrellas.

(a) Write a linear function U(p) that predicts the number of umbrellas sold based on the price p.
(b) How many umbrellas do they expect to sell if they raise the price to $20?

Answer 1

The answer is below

Explanation:

a) A linear function shows the relationship between variables. A linear equation is in the form: y = mx + b, where m is the rate of change, b is the value of y when x = 0, y is the dependent variable and x is an independent variable.

Let U represent the number of umbrellas and p the price of the umbrella. At $10 the number of umbrella sold is 18000, it can be represented as (10, 18000) i.e. (p, U)

At $16 the number of umbrella sold is 9000, it can be represented as (16, 9000).

The linear function is determined using:

`U-U_1=(U_2-U_1)/(p_2-p_1)(p-p_1)`

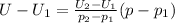
Given (10, 18000) and (16, 9000):

`U-U_1=(U_2-U_1)/(p_2-p_1)(p-p_1)\\\\U-18000=(9000-18000)/(16-10)(p-10)\\\\U-18000=-1500(p-10)\\\\U-18000=-1500p+15000\\\\U=-1500p+15000+18000\\\\U=-1500p+33000\\\\U(p)=-1500p+33000`

b) At p = $20, The number of umbrella is:

U(20) = -1500(20)+33000

U(20) = 3000 umbrella