Let's take this problem step-by-step:
Let's first set up some variables:
- c: # of children
- a: # of adults
Let's examine the information given:
- Elevator can hold a maximum of 1500 pounds
⇒ average child is 75 pounds
⇒ average adult is 150 pounds
⇒ therefore:
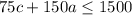
- Elevator can fit no more than 14 people
⇒ therefore:
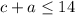
Let's graph the equations:
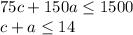
⇒ look at the image attached
The point at which the two graphs intersect:
⇒ is the solution that represents the amount of children and adults and
their combine weight
With the horizontal axis being the # of children and vertical axis being the # of adults:
⇒ the solution is 8 children and 6 adults
Answer: 8 children and 6 adults
Hope that helped!