Question

el área de un terreno rectangular es de 616 metros cuadrados si el largo del terreno mide 6 m más que el ancho cuáles son sus dimensiones​

Answer 1

length 28, width 22

Explanation:

28 - 22 = 6, 28 x 22 = 616

Answer 2

The rectangular plot has a width of 22 meters and a length of 28 meters, as determined by solving the equation for the given area of 616 square meters, where the length is 6 meters more than the width.

Let's denote the width of the rectangular plot as w meters. According to the given information, the length is 6 meters more than the width, so the length is w + 6 meters.

The area A of a rectangle is given by the formula:

`\[ A = \text{length} * \text{width} \]`

In this case, we know that the area is 616 square meters:

`\[ 616 = (w + 6) * w`

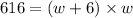
Now, let's solve for w:

`\[ w^2 + 6w - 616 = 0 \]`

We can factor this quadratic equation:

(w + 28)(w - 22) = 0

So, w = 22 or w = -28. Since the width cannot be negative, the width is 22 meters.

Therefore, the dimensions of the rectangular plot are:

- Width: 22 meters

- Length: 22 + 6 = 28 meters

The probable question may be:

The area of ​​a rectangular plot of land is 616 square meters. If the length of the plot measures 6 m more than the width, what are its dimensions?