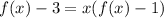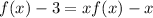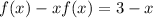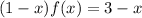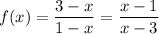Question

D. If f¹(x+2) =X-1/x+1x not equal to 1 then find f(x) and f¹(4).​

Answer 1

I assume by f¹, you actually mean f⁻¹ as in the inverse of f. I also assume you are asked to find f(x) (as in the inverse of f⁻¹) and f⁻¹(4).

Given that

`f^(-1)(x+2) = (x-1)/(x+1)`

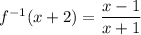
with x ≠ 1, we can find f⁻¹(x) by replacing x + 2 with x :

`f^(-1)(x + 2) = (x-1)/(x+1) = ((x+2) - 3)/((x + 2) - 1) \implies f^(-1)(x) = (x-3)/(x-1)`

Then when x = 4, we have

`f^(-1)(4) = (4-3)/(4-1) = \frac13`

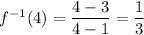
Of course, we also could have just substituted x = 2 into the definition of f⁻¹(x + 2) :

`f^(-1)(4) = f^(-1)(2+2) = (2-1)/(2+1) = \frac13`

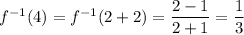
To find f(x), we fall back to the definition of an inverse function:

`f^(-1)\left(f(x)\right) = x`

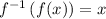
Then by definition of f⁻¹, we have

`f^(-1)\left(f(x)\right) = (f(x)-3)/(f(x)-1) = x`

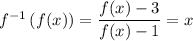
Solve for f :

`f(x) - 3 = x (f(x) - 1)`

`f(x) - 3 = x f(x) - x`

`f(x) - x f(x) = 3 - x`

`(1 - x) f(x) = 3-x`

`f(x) = (3-x)/(1-x) = (x-1)/(x-3)`