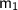 = 8kg
= 8kg
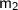 = 4kg
= 4kg
g = 10m/s²
→Acceleration of block
→Tension in strings
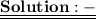
Let, the two equation will be ,
 8g - T = 8a ..... Equation no (1)
8g - T = 8a ..... Equation no (1)
 T - 4g = 4a ..... Equation no (2)
T - 4g = 4a ..... Equation no (2)
Putting the value of equation no (1) and (2) we get,
 4g = 12a
4g = 12a
 4 × 10 = 12a
4 × 10 = 12a
 40 = 12a
40 = 12a
 a =
a =
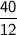
 a =
a =

Again, we have to putting the value of a in the equation no (2) we get,
 T - 4g = 4 ×
T - 4g = 4 ×

 T - 4 × 10 = 4 ×
T - 4 × 10 = 4 ×

 T - 40 =
T - 40 =
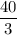
 T =
T =
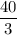 + 40
+ 40
 T =
T =
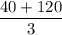
 T =
T =
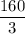 N
N
 Acceleration of block =
Acceleration of block =
 m/s².
m/s².
And, Tension in strings =
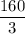 N
N