Question

Please Help!
The following is an isosceles trapezoid, find m < J

Answer 1

The measure of angle J is 44°

Explanation:

Isosceles Trapezoid

The isosceles trapezoid has its non-parallel sides of the same length, thus the angles they form with their respective parallel sides are congruent.

It means: Angle J is congruent with angle K and angle M is congruent with angle L

The sum of angles in a trapezoid is 180*(4-2)=360°

Thus:

`m\angle J+m\angle K+m \angle M+m\angle L = 360^\circ`

`7x+2+7x+2+25x-14+25x-14=360`

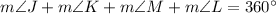
Joining like terms:

`64x-24=360`

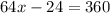
Adding 24:

`64x=384`

Dividing by 64:

x=6°

Thus:

`m\angle J = 7*6+2=44^\circ`

`m\angle M = 25*6-14=136^\circ`

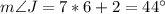
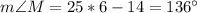
The measure of angle J is 44°