Note: Consider "÷" sign instead of "=".
Given:
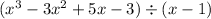
To find:
The quotient.
Solution:
We have,
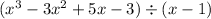
It can be written as
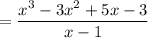
Splitting the middle terms, we get

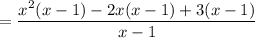
Taking out the common factor (x-1), we get
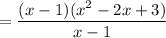
Cancel the common factors.
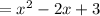
The quotient is
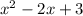 .
.
Therefore, the correct option is D.