Question

If the endpoints of AB are at (-4, 5) and (2, -7), what is the length of AB?​

Answer 1

The length of AB is
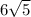`6√(5)` units

Explanation:

The rule of the distance between two points (x1, y1) and (x2, y2) is

• 
  `d=\sqrt{(x2-x1)^(2)+(y2-y1)^(2)}`

∵ The endpoints of AB are (-4, 5) and (2, -7)

→ Let point (-4, 5) be (x1, y1) and point (2, -7) be (x2, y2)

∴ x1 = -4 and y1 = 5

∴ x2 = 2 and y2 = -7

→ Substitute them in the rule above to find the length of AB

∵
`AB=\sqrt{(2--4)^(2)+(-7-5)^(2) }=\sqrt{(2+4)^(2)+(-12)^(2)}`

∴
`AB=\sqrt{(6)^(2)+144}=√(36+144)`

∴
`AB=√(180)=6√(5)`

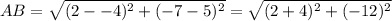
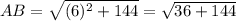
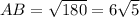
∴ The length of AB is
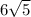`6√(5)` units