Given:
Y-intercept of exponential function is 8.
It contains the point (3,64).
To find:
The exponential function that describes the graph.
Solution:
The general form of an exponential function is
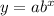 ...(i)
...(i)
where, a is initial value or y-intercept and b is growth factor.
Since, y-intercept is 8, therefore, a=8.
Put a=8 in (i).
 ...(ii)
...(ii)
It contains the point (3,64). Put x=3 and y=64.
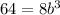
Divide both sides by 8.
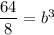
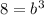
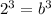
On comparing both sides, we get
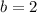
Put b=2 in (ii).
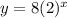
The functions form of this equation is

Therefore, the required function is
 .
.