Answer:
The probability that 24 or more from this sample will be female is 0.2643.
Explanation:
Define X as the number of female students.
The random variable X follows a Binomial distribution with parameters n = 40 and p = 0.55.
But the sample selected is too large and the probability of success is close to 0.50.
So a Normal approximation to binomial can be applied to approximate the distribution of X if the following conditions are satisfied:
1. np ≥ 10
2. n(1 - p) ≥ 10
Check the conditions as follows:

Thus, a Normal approximation to binomial can be applied.
So, X follows normal distribution with mean and standard deviation:

Compute the probability that 24 or more from this sample will be female as follows:
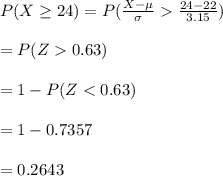
Thus, the probability that 24 or more from this sample will be female is 0.2643.