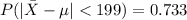Answer:
The probability is
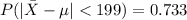
Explanation:
From the question we are told that
The mean mileage of a tire is
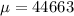
The standard deviation is
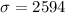
The sample size is n = 209
Generally the standard error of mean is mathematically represented as
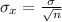
=>

=>

Generally the probability that the sample mean would differ from the population mean by less than 199 miles is mathematically represented as
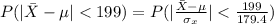
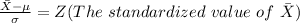
=>
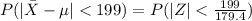
=>
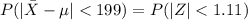
=>
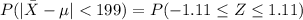
=>
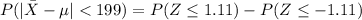
From the z table the area under the normal curve to the left corresponding to 1.11 and -1.11 is
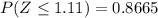
and
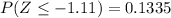
So
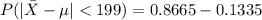
=>