Question

(7)Figure 4 shows three charges: Q₁, Q₂ and Q3 . Determine the net force (Fnet) acting on Q3. (Hint: Draw a free body diagram of the forces to assist you with the calculation.)

(8)Figure 5 shows three charges arranged in a right angled formation.

(8.1)Draw a free body diagram of the forces that act on the -0,03 uC charge.

(8.2)Calculate each force that acts on the -0,03 uC charge.

(8.3) Find the magnitude and direction of the net force that acts on the 0,03 μC charge with the aid of a diagram and by calculations.

Help Please.​

Answer 1

Remember Coulomb's law: the magnitude of the electric force F between two stationary charges q₁ and q₂ over a distance r is

`F = (kq_1q_2)/(r^2)`

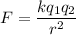
where k ≈ 8,98 × 10⁹ kg•m³/(s²•C²) is Coulomb's constant.

8.1. The diagram is simple, since only two forces are involved. The particle at Q₂ feels a force to the left due to the particle at Q₁ and a downward force due to the particle at Q₃.

8.2. First convert everything to base SI units:

0,02 µC = 0,02 × 10⁻⁶ C = 2 × 10⁻⁸ C

0,03 µC = 3 × 10⁻⁸ C

0,04 µC = 4 × 10⁻⁸ C

300 mm = 300 × 10⁻³ m = 0,3 m

600 mm = 0,6 m

Force due to Q₁ :

`F_(Q_2/Q_1) = (k (6 * 10^(-16) \,\mathrm C))/((0,3 \, \mathrm m)^2) \approx \boxed{6,0 * 10^(-5) \,\mathrm N} = 0,06 \,\mathrm{mN}`

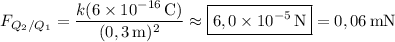
Force due to Q₃ :

`F_(Q_2/Q_3) = (k (12 * 10^(-16) \,\mathrm C))/((0,6 \, \mathrm m)^2) \approx \boxed{3,0 * 10^(-5) \,\mathrm N} = 0,03 \,\mathrm{mN}`

8.3. The net force on the particle at Q₂ is the vector

`\vec F = F_(Q_2/Q_1) \, \vec\imath + F_(Q_2/Q_3) \,\vec\jmath = \left(-0,06\,\vec\imath - 0,03\,\vec\jmath\right) \,\mathrm{mN}`

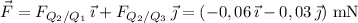
Its magnitude is

`\|\vec F\| = \sqrt{\left(-0,06\,\mathrm{mN}\right)^2 + \left(-0,03\,\mathrm{mN}\right)^2} \approx 0,07 \,\mathrm{mN} = \boxed{7,0 * 10^(-5) \,\mathrm N}`

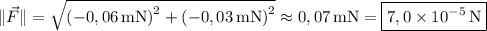
and makes an angle θ with the positive horizontal axis (pointing to the right) such that

`\tan(\theta) = (-0,03)/(-0,06) \implies \theta = \tan^(-1)\left(\frac12\right) - 180^\circ \approx \boxed{-153^\circ}`

where we subtract 180° because
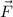`\vec F` terminates in the third quadrant, but the inverse tangent function can only return angles between -90° and 90°. We use the fact that tan(x) has a period of 180° to get the angle that ends in the right quadrant.