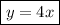Question

2. Find the equation of a line
a) With a slope of 4, passing through the origiin​

Answer 1

3x

Explanation:

Answer 2

`y=4x`

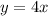
Explanation:

Equation of the line

A line can be expressed in many forms.

The equation of the line in slope-intercept form is:

y=mx+b

Being m the slope and b the y-intercept.

The point-slope form of the equation of a line is:

y-k=m(x-h)

Where m is the slope and (h,k) is a point through which the line passes.

The equation of a line passing through points (x1,y1) and (x2,y2) can be found as follows:

`\displaystyle y-y_1=(y_2-y_1)/(x_2-x_1)(x-x_1)`

We are required to find a line with slope m=4 passing through the origin (0,0).

Using the point-slope form:

y-0=4(x-0)

Simplifying

`\boxed{y=4x}`