Question

A bridge is sketched in the coordinate plane as a parabola represented by the equation h=40-0.01x2, where h refers to the height, in feet, of the bridge at a location of x feet from the middle of the bridge. The bridge starts and ends at the x-intercepts of the parabola. What is the length of this bridge?

Answer 1

The length of the bridge is 126.492 feet.

Explanation:

Let
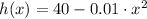`h(x) = 40-0.01\cdot x^(2)`, where
`x` is the position from the middle of the bridge, measured in feet, and
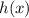`h(x)` is the height of the bridge at a location of x feet, measured in feet. In this case, the length of the bridge is represented by the distance between the x-intercepts of the parabola, which we now find by factorization:

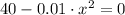`40-0.01\cdot x^(2) = 0` (Eq. 1)

`x^(2) = (40)/(0.01)`

`x =\pm \sqrt{(40)/(0.01) }`

`x = \pm 63.246\,ft`

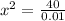
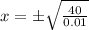
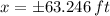
Given that the parabola is symmetrical with respect to y-axis, then the length is two times the magnitude of the value found above, that is:

`l = 2\cdot (63.246\,ft)`

`l = 126.492\,ft`

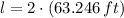
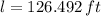
The length of the bridge is 126.492 feet.