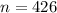Question

A software developer wants to know how many new computer games people buy each year. Assume a previous study found the variance to be 1.44. She thinks the mean is 6 computer games per year. What is the minimum sample size required to ensure that the estimate has an error of at most 0.15 at the 99% level of confidence

Answer 1

The sample size is
`n = 426`

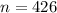
Explanation:

From the question we are told that

The population variance is
`\sigma^2 = 1.44`

The mean is
`\= x = 6`

The margin of error is E = 0.15

Generally the standard deviation is mathematically represented as

`\sigma = √(\sigma^2 )`

=>
`\sigma = √(1.44 )`

=>
`\sigma = 1.2`

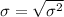
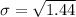
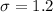
From the question we are told the confidence level is 99% , hence the level of significance is

`\alpha = (100 - 99 ) \%`

=>
`\alpha = 0.01`

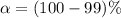
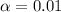
Generally from the normal distribution table the critical value of
`(\alpha )/(2)` is

`Z_{(\alpha )/(2) } =  2.58`

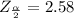
Generally the sample size is mathematically represented as

`n = [\frac{Z_{(\alpha )/(2) } *  \sigma }{E} ] ^2`

=>
`n = [(2.58 * 1.2 )/(0.15) ] ^2`

=>
`n = 426`