Question

g An 85.2-mH inductor and a 5.27-pF capacitor are connected in series with a generator whose frequency is 343 Hz. The rms I voltage across the capacitor is2.42V. Determine the rms voltage across the inducton

Answer 1

The rms voltage across the inductor is 5.05 x 10⁻⁶ V.

Step-by-step explanation:

Given;

inductance of the inductor, L = 85.2 mH = 85.2 x 10⁻³ H

capacitance of the capacitor, C = 5.27 pF = 5.27 x 10⁻¹² F

frequency of the generator, f = 343 Hz

Since the inductor, and capacitor are connected in series, same current will pass through them.

The current through them is given by;

`I_(rms) = (V_c_((rms)))/(X_c) = (V_l_((rms)))/(X_L)`

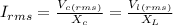
where;

Xc and XL are capacitive and inductive reactance respectively;

`(V_c_((rms)))/((1)/(2\pi fC) ) = (V_l_((rms)))/(2\pi fL)\\\\(V_c_((rms))(2\pi fC))/(1) = (V_l_((rms)))/(2\pi fL)\\\\V_l_((rms)) = V_c_((rms))(2\pi fC)(2\pi fL)\\\\V_l_((rms)) =V_c_((rms))(4\pi^2 f^2 CL)\\\\V_l_((rms)) = (2.42)(4\pi^2)(343)^2(5.27*10^(-12))(85.2*10^(-3))\\\\V_l_((rms)) = 5.05 *10^(-6) \ V`

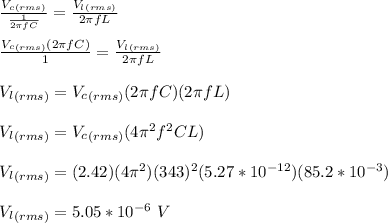
Therefore, the rms voltage across the inductor is 5.05 x 10⁻⁶ V.