Answer: The speed of red horse = 44 feet per second and the speed of black horse = 35.2 feet per second.
Explanation:
Given: A red horse and a black horse raced on a 1-mile-long circular racetrack.
Time taken by red horse = 120 seconds
Time taken by black horse = 150 seconds
Speed =

So, speed of red horse =
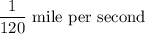
Since 1 mile = 5280 feet
Speed of red horse =
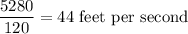
Similarly,
Speed of black horse =
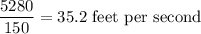
Hence, the speed of red horse = 44 feet per second and the speed of black horse = 35.2 feet per second.