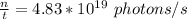Answer:
The value is
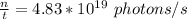
Step-by-step explanation:
From the question we are told that
The power rating of the bulb is
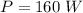
The frequency is

The percentage of the input power that is emitted as visible light is
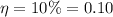
Generally the amount of power emitted as visible light is mathematically represented as
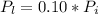
=>
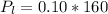
=>
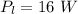
Generally the amount of energy emitted as light is mathematically represented as
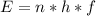
Here n is the number of photon , h is the Planks constant with value

Generally this power emitted as visible light is mathematically represented as
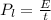
=>
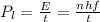
=>
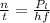
=>
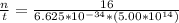
=>