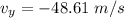Answer:
The value is
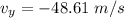
Step-by-step explanation:
From the question we are told that
The horizontal speed is
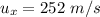
The horizontal distance is

Generally the time taken by the hot magma in air before landing is mathematically represented as
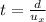
=>
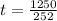
=>
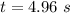
Generally the initial vertical velocity of the magma when it was lunched is
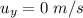
Then the final velocity of the magma when it hits the ground is mathematically represented s
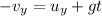
Here the negative sign mean that the direction of the velocity is towards the negative y -axis
So
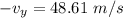
=>