Question

From the side of a hill wai-kin looks up at the top of a nearby vertical cliff at an angle of elevation of 36 degrees and looks down at the bottom of the cliff at an angle of depression of 47 degrees. Wai-kin and the cliff are 490 meters apart.

Answer 1

881.5 meters.

Explanation:

The horizontal plane at the level of wai-kin's eyes divide the height of the cliff into two parts. Let the upper part be represented by x and the lower part be represented by y.

The height of the cliff = x + y

Applying the appropriate trigonometry functions.

To determine the value of x;

Tan θ =
`(opposite)/(adjacent)`

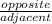
Tan
`36^(o)` =
`(x)/(490)`

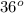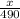
⇒ x = 490 x Tan
`36^(o)`

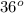
= 490 x 0.7265

= 355.985

x = 356 meters

To determine the value of y;

Tan θ =
`(opposite)/(adjacent)`

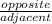
Tan
`47^(o)` =
`(y)/(490)`

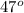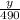
⇒ y = 490 x Tan
`47^(o)`

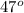
= 490 x 1.0724

= 525.476

y = 525.5 meters

Therefore,

The height of the cliff = x + y

= 355.985 + 525.476

= 881.461

The height of the cliff is 881.5 meters.