Answer:

Explanation:
To solve a quadratic equation by completing squares we need to recall these basic algebra relations called the square of a binomial:
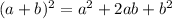
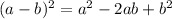
We can use them from right to left to factor trinomials:
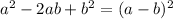
We need to solve the equation:
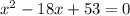
Subtracting 53:
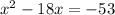
The first two terms of the left side of the equation are the first two terms of the trinomial given in the formula, having:
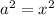
Or, equivalently:

Also:
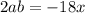
Since a=x

Simplifying:
b=-9
We need to complete the square by adding
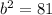
Adding 81:
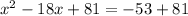
Factoring and operating:
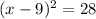
Since 28=4*7
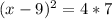
Taking square roots:
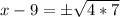
Separating the square root of 4:
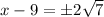
Solving:
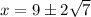
The two solutions are:
