Question

A train stops at two stations A and B. It accelerates from rest from station A to a speed of 144 km h^-1 in 3 minutes and maintains this speed for 10 minutes. It then decelerates for 2 minutes and comes to rest at station B. Find the total distance between A and B.

Show work please, really need the help.

Answer 1

The total distance between A and B is 30 km

Step-by-step explanation:

The given information are;

The time duration of the acceleration of the train from station A = 3 minutes = 0.05 hours

The speed attained by the train after the acceleration = 144 km/h

The time duration the train maintains the speed = 10 minutes = 0.1
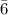`\bar 6` hours

The time duration in which the train decelerates to station B = 2 minutes = 0.0
`\bar 3` hours

The equation of motion required are;

The initial acceleration, a = (144 - 0)/0.05 = 2,880 km/h²

The distance covered, s = u·t + 1/2·a·t²

Where;

u The initial velocity = 0

∴ s₁ = 0 × 0.05 + 1/2 × 2880 × 0.05² = 3.6 km

s₁ = 3.6 km

The distance, s₂ the train covers at the constant speed 144 km/h for 10 minutes (1/6 hours) is given as follows;

s₂ = Velocity Time = 144 × 1/6 = 24 km

s₂ = 24 km

The deceleration, a₂ that brings the train to a stop in 2 minutes (1/30 hours) is given as follows;

a₂ = (0 - 144)/(1/30) = -4320 km/h²

The distance covered, s₃ by the train as it decelerates to rest from the initial constant speed is given as follows;

s₃ = u·t + 1/2·a·t²

Where;

u = The initial velocity =144 km/h

We have;

s₃ = 144×1/30 - 1/2 × 4320 × (1/30)² = 2.4

s₃ = 2.4 km

The total distance between A and B, s = s₁ + s₂ + s₃ = 3.6 + 24 + 2.4 = 30 km

The total distance between A and B = 30 km.