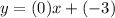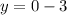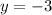Question

What is the equation of the line shown in this graph?

A function graph of a line with two points (-2,-3) and (3,-3) with an x axis of negative five to five and a y axis of negative five to five

Enter your answer in the box.

Answer 1

Explanation:

Hope this helps Amy~

Answer 2

`y = -3`

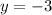
Explanation:

Given:

(-2,-3);

(3,-3)

Required:

Equation of the line that passes through both points on a graph

SOLUTION:

Equation of the line can be represented in slope-intercept form as
`y = mx + b`

Where,

m = slope of the line. Since the line is horizontal, the slope is 0 as with all horizontal lines. Therefore, m = 0

b = y-intercept value, which is the point at which the line intercepts the y-axis. the line intercepts the y-axis at -3. Therefore, b = -3.

Now, substitute m = 0 and b = -3 into
`y = mx + b`

`y = (0)x + (-3)`

`y = 0 - 3`

`y = -3`