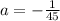Answer:
No she isn't. The value of
 is
is
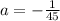
Explanation:
We know that all quadratic equation can be written in the following way :
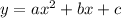 (I)
(I)
Where
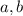 and
and
 are real numbers.
are real numbers.
I will attach a drawing with the quadratic graph to understand the situation.
We know by looking at the drawing and analyzing it that the parabola passes through the points :
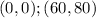 and
and

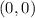 because we put our coordinates origin there.
because we put our coordinates origin there.
 because that's where the golf hole is.
because that's where the golf hole is.
And
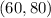 because we know that its highest point reaches 80 feet up in the air at the middle of the distance between its roots (property of a negative parabola).
because we know that its highest point reaches 80 feet up in the air at the middle of the distance between its roots (property of a negative parabola).
Finally, we work with the three points and the equation (I) in order to find the values of
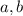 and
and
 ⇒
⇒
The parabola passes through
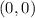 ⇒
⇒
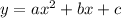 ⇒
⇒
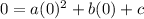 ⇒
⇒

The parabola passes through
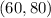 ⇒
⇒
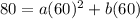 ⇒
⇒
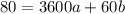 (II)
(II)
The parabola passes through
 ⇒
⇒
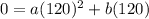 ⇒
⇒
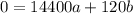
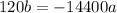
 (III)
(III)
Now if we use (III) in (II) ⇒
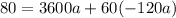
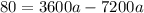

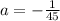 ⇒
⇒
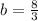
Finally the equation of the parabola is

Where the value of
 is
is