Given:
The value of the function g(x) is −2 when x=−5 and is 5.7 when x=6.
To find:
The equation of the function.
Solution:
The value of the function g(x) is −2 when x=−5. It means the graph of function passes through (-5,-2).
The value of the function g(x) is 5.7 when x=6. It means the graph of function passes through (6,5.7).
The equation of function g(x) that passes through (-5,-2) and (6,5.7) is

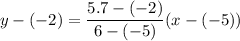

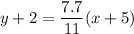
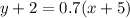
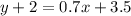
Subtract 2 from both sides.
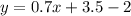
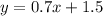
Therefore, the equation of function g(x) is
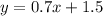 .
.