Answer:
The correct option is; A.
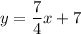
Explanation:
The given equation is 4·y - 7·x = 8
The coordinates of the point the line passes = (-4, 0)
By dividing the given equation by 4, we have;
4·y/4 - 7/4·x = 8/4
Which gives;
y - 7/4·x = 2
y = 7/4·x + 2
Which is the equation of a straight line in slope and intercept form, y = m·x + c, with the slope, m = 7/4, and the y-intercept = 2
Given that the required line is parallel to the graph of the equation, 4·y - 7·x = 8, the slopes of both equations are equal we have;
Slope of required line = 7/4, point on the line = (-4, 0), which gives the following point and slope form equation of a straight line;
y - 0 = 7/4 × (x - (-4))
y = 7/4·x + 7/4 × 4 = 7/4·x + 7
The equation of the line is therefore;
y = 7/4·x + 7