Answer: 0.2501
Explanation:
Given : The proportion of the bulbs in the lot are defective = 20%= 0.20
Sample size : n =15
Let x be the binomial variable that represents the defective bulbs.
Binomial probability formula :
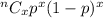
The probability that exactly 3 bulbs from the sample are defective : P(X=3)
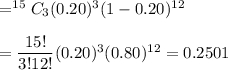
Required probability = 0.2501