Answer:
The separation in meters on the screen between the bright fringes of the two interference patterns is 1.08 × 10⁻⁴ m
Step-by-step explanation:
Here is the complete question:
In a double-slit experiment, the distance between slits is 5.6 mm and the slits are 0.18 m from the screen. Two interference patterns can be seen on the screen: one due to light of wavelength 410 nm, and the other due to light of wavelength 660 nm. What is the separation in meters on the screen between the third-order (m = 3) bright fringes of the two interference patterns?
Step-by-step explanation:
For a bright fringe, the distance
 of the bright fringe can be determined from
of the bright fringe can be determined from
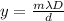
Where
 is the order
is the order
 is the wavelength
is the wavelength
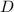 is the distance from the screen
is the distance from the screen
 is the distance between the slits
is the distance between the slits
From the question,
 = 5.6 mm = 5.6 × 10⁻³ m
= 5.6 mm = 5.6 × 10⁻³ m
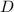 = 0.81 m
= 0.81 m
m = 3
For the first interference pattern,
 = 410 nm = 410 × 10⁻⁹ m
= 410 nm = 410 × 10⁻⁹ m
∴
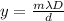 becomes
becomes
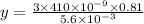
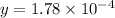 m
m
For the second interference pattern,
 = 660 nm = 660 × 10⁻⁹ m
= 660 nm = 660 × 10⁻⁹ m
∴

 m
m
Now, the separation between the bright fringes of the two interference patterns will be the difference in the distances of the bright fringes for the two interference patterns. That is
2.86 × 10⁻⁴ m - 1.78 × 10⁻⁴ m = 1.08 × 10⁻⁴ m
Hence, the separation in meters on the screen between the bright fringes of the two interference patterns is 1.08 × 10⁻⁴ m.