The given series can be condensed to
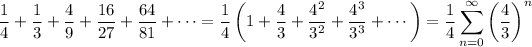
which is a geometric series with ratio 4/3. Since this ratio is larger than 1, the sequence of partial sums of the series diverges, so the infinite series also diverges.
We can also just the n-th term test:
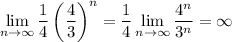
since 4ⁿ > 3ⁿ for all n > 0.