Answer:
The correct option is;
∠ADB ≅ ∠CDB,
 ≅
≅
 and
and
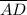 ≅
≅
 . So ΔABD ≅ ΔCDB by SAS
. So ΔABD ≅ ΔCDB by SAS
Explanation:
The given parameters are;
The polygon given = Quadrilateral
The diagonal of the quadrilateral = BD
The length of the side AD = (2×y + 5) units
The length of the side CD = (y + 10) units
The given measure of ∠ABD = (2 × y + 25)°
The given measure of ∠CBD = 35°
When y = 5, we have by substituting the value of y into the equations representing the dimensions of the quadrilateral ABCD;
The length of the side AD = 2 × y + 5 = 2×5 + 5 = 15 units
∴ The length of the side AD = 15 units
The length of the side CD = (y + 10) units = (5 + 10) units = 15 units
∴ The length of the side CD = 15 units
The measure of ∠ABD = (2 × y + 25)° = (2 × 5 + 25)° = 35°
The measure of ∠ABD = 35°
Therefore;
∠ADB ≅ ∠CDB by substitution
 ≅
≅
 by reflexive property
by reflexive property
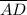 ≅
≅
 by substitution
by substitution
∴ ΔABD ≅ ΔCBD by Side-Angle-Side rule of congruency