Question

Assume that adults were randomly selected for a poll. They were asked if they​ "favor or oppose using federal tax dollars to fund medical research using stem cells obtained from human​ embryos." Of those​ polled, were in​ favor, were​ opposed, and were unsure. A politician claims that people​ don't really understand the stem cell issue and their responses to such questions are random responses equivalent to a coin toss. Exclude the subjects who said that they were​ unsure, and use a significance level to test the claim that the proportion of subjects who respond in favor is equal to . What does the result suggest about the​ politician's claim

Answer 1

Answer:c : there is no sufficient evedince to support the claim that more than 50% of adults are opposed to federal tax dollars being used for foreign aid

Answer 2

Complete Question

Assume that adults were randomly selected for a poll. They were asked if they "favor or oppose using federal tax dollars to fund medical research using stem cells obtained from human embryos." Of those polled, 487 were in favor, 398 were opposed, and 116 were unsure. A politician claims that people don't really understand the stem cell issue and their responses to such questions are random responses equivalent to a coin toss. Exclude the 116 subjects who said that they were unsure, and use a 0.05 significance level to test the claim that the proportion of subjects who respond in favor is equal to 0.5. What does the result suggest about the politician's claim?

a) Identify the null and alternative hypotheses for this test.

b) The test statistic for this hypothesis test is?

c) The P-value for this hypothesis test is?

d) Identify the conclusion for this hypothesis test.

e) What does the result suggest about the politician's claim?

Answer:

a

The null hypothesis is
`H_o : p = 0`

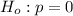
The alternative hypothesis
`H_a: p \\e 0.5`

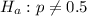
b

The test statistics
`z = 2.993`

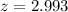
c

The
`p-value = 0.002762`

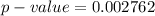
d

The decision rule is

Reject the null hypothesis

The conclusion is

There is no sufficient evidence to show that that people don't really understand the stem cell issue and their responses to such questions are random responses equivalent to a coin toss

e

The politicians claim is false

Explanation:

From the question we are told that

The number of people that where in favor is k = 487

The number that opposed is u = 398

The number that where unsure r = 116

The level of significance is
`\alpha = 0.05`

given the number those who where unsure is excluded then the sample size is

`n = k + u`

=>
`n = 487 + 398`

=>
`n = 885`

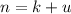
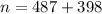
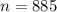
Generally the sample proportion of those who where in favor is mathematically represented as

`\^ p = (k)/(n)`

=>
`\^ p = (487)/(885)`

=>
`\^ p = 0.5503`

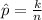
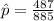
From the question we are told that a politician claims that people don't really understand the stem cell issue and their responses to such questions are random responses equivalent to a coin toss , hence the population proportion of those who are in favor is

p = 0.5 (equivalent to probability of heads or tails )

The null hypothesis is
`H_o : p = 0`

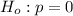
The alternative hypothesis
`H_a: p \\e 0.5`

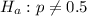
Generally the test statistics is mathematically as

`z = \frac{ \^ p - p}{\sqrt{(p(1 - p) )/(n) } }`

=>
`z = \frac{ 0.5503 - 0.5}{\sqrt{(0.5(1 - 0.5) )/(885) } }`

=>
`z = 2.993`

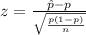
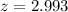
Generally the p-value is mathematically represented as

`p-value = 2 * P( z > 2.993)`

From the z table the area under the normal curve corresponding to 2.993 to the right is

`P( z > 2.993) = 0.0013812`

=>
`p-value = 2 * 0.0013812`

=>
`p-value = 0.002762`

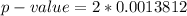
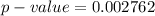
From the value obtained we see that the
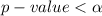`p-value < \alpha` hence

The decision rule is

Reject the null hypothesis

The conclusion is

There is no sufficient evidence to show that that people don't really understand the stem cell issue and their responses to such questions are random responses equivalent to a coin toss

Now looking at the result obtained and the conclusion made , it means that the politicians claim is false