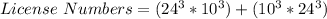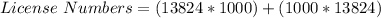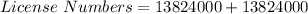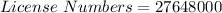Question

In the state of Texas during the years 1984 and 1985, all license plate identifiers were formed using either three uppercase English letters (except that O and I were not used) followed by three digits, or by three digits followed by three letters (except O and I). How many distinct license plates were possible using this policy

Answer 1

`License\ Numbers = 27648000`

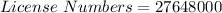
Explanation:

Given

Alphabets (exception of O and I) = 24

Digits = 10

Required

Determine the number of license plates

From the question, we understand that the arrangement could be:

Arrangements = (Alphabets and Digits) or (Digits and Alphabets)

In both cases:

The first alphabet could be any of the 24 usable alphabets

The second could be any of the 24

The third could also be any of the 24

So, the alphabets can be arranged in: 24³ ways

The 1st digit could be any of the 10

The 2nd could be any of the 10

The 3rd could also be any of the 10

So, the digits can be arranged in: 10³ ways

Number of License Plates is then calculated as thus:

`License\ Numbers = (24^3 * 10^3) + (10^3 * 24^3)`

`License\ Numbers = (13824 * 1000) + (1000 * 13824)`

`License\ Numbers = 13824000 + 13824000`

`License\ Numbers = 27648000`